Dec 08, · Like sin 2 θ cos 2 θ = 1 and 1 tan 2 θ = sec 2 θ etc Such identities are identities in the sense that they hold for all value of the angles which satisfy the given condition among them and they are called conditional identities Trigonometric Identities With ExamplesSin 2 (x) cos 2 (x) = 1 tan 2 (x) 1 = sec 2 (x) cot 2 (x) 1 = csc 2 (x) sin(x y) = sin x cos y cos x sin y cos(x y) = cos x cosy sin x sin yVerify the identity \cos ^{2} x\tan ^{2} x=2\sin ^{2} x\sec ^{2} x Video Transcript Well, let's no analyze these identity Let's prove this identity
Integrate Sec 2x Method 2
Tan^2 sec^2 identity
Tan^2 sec^2 identity-Math Cheat Sheet for Trigonometry One Time Payment $1299 USD for 2 months Weekly Subscription $199 USD per week until cancelled Monthly Subscription $699 USD per month until cancelled Annual Subscription $2999 USD per year untilExample Verify the given identity \(\cos 2 x=\frac{1\tan ^{2} x}{1\tan ^{2} x}\)
Trig Identity Sec2x Minus Tan2x T10 Youtube
Prove the following trigonometric identities sec^6 θ = tan^6 θ 3tan^2 θsec^2 θ 1 ← Prev Question 0 votes 5 views asked 12 hours ago in Trigonometry by Gaatrika (173k points) Prove the following trigonometric identities sec 6 θ = tan 6 θ 3tan 2 θsec 2 θ 1 trigonometric identities;Aug 05, 15 · Simplify tan^2 x sec^2 Ans 1 Use trig identity 1 tan^2 x = sec^2 x tan^2 x sec^2 x = 1View Basic_Identities__Day_2docx from MATH 1325 at Lone Star College System Basic Identities Day 2 NameClass PeriodDate sin = 1 csc = csc cos = 1 sec tan = sin tan
Jan 08, 18 · True Start with the well known pythagorean identity sin^2x cos^2x = 1 This is readily derived directly from the definition of the basic trigonometric functions sin and cos and Pythagoras's Theorem Divide both side by cos^2x and we get sin^2x/cos^2x cos^2x/cos^2x = 1/cos^2x tan^2x 1 = sec^2x tan^2x = sec^2x 1 Confirming that the result is an identityFree math lessons and math homework help from basic math to algebra, geometry and beyond Students, teachers, parents, and everyone can find solutions to their math problems instantlyBecause the two sides have been shown to be equivalent, the equation is an identity tan2(2x)sin2(2x) cos2(2x) = sec2 (2x) tan 2 (2 x) sin 2 (2 x) cos 2 (2 x) = sec 2 (2 x) is an identity
Prove the following trigonometric identities tan 2 A cot 2 A = sec 2 A cosec 2 A − 2 Advertisement Remove all ads Solution Show Solution In the given question, we need to prove tan 2 A cot 2 A = sec 2 A cosec 2 A − 2 Now using `tan theta = sin theta/cos theta` and `cot theta = cos theta/sin theta` in LHS we getJul 03, · Working problems involving doubleangle identities is very similar to the other identities we've worked with previously you just have more identities to choose from!Nov 27, 13 · = sec 2 A (sec 2 A 1) = (1 tan 2 A) (tan 2 A) = tan 2 A tan 4 A= RHS Hence sec 4 A sec 2 A = tan 4 A tan 4 A Answered by 27th Nov, 13, 0312 AM Concept Videos Introduction to Trigonometric Identities Introduction to Trigonometry, Introduction to Trigonometric Identities Practice Test Webinar Pre Board Assessment
Sample Problems Cos 2 X Tan2 X Tan 2 Csc 2 Tan Sec X Tan X Cos X Sin 4 X Cos 4 X 1 2 Cos 2 X Pdf Free Download
Ex 7 3 15 Integrate Tan3 2x Sec 2x Class 12 Ncert Ex 7 3
Apr , · Similarly, to derive the Pythagorean identity tan 2 x 1 = sec 2 x, divide through by cos 2 x and simplify sin 2 x cos 2 x cos 2 x cos 2 x = 1 cos 2 x tan 2 x 1 = sec 2 x Examples Example 1 Earlier, you were asked if tan 2 x cot 2 x = 1 is a legitimate identity Cofunctions are not always connected directly through a Pythagorean(2) 1 = cot(a) The left side is more complicated so start there tan(a) sec2(X) 1 = tan(x) Use a Pythagorean identity on the denominator = tan(3) Now you have = 2 Cancel = Now you have 3 Use a reciprocal identity This problem has been solved!Didn't find what you were looking for?
Important Questions For Class 10 Maths Chapter 8 Introduction To Trigonometry Learn Cbse
10 4 Integration Of Powers Of Trigonometric Functions
Free trigonometric identities list trigonometric identities by request stepbystep This website uses cookies to ensure you get the best experience =tan^{2} (x/2) en Related Symbolab blog posts Spinning The Unit Circle (Evaluating Trig Functions ) If you've ever taken a ferris wheel ride then you know about periodic motion, you goUsing the identity 1 tan2 = sec2 we nd sec2 1 sec 2 = 1 tan2 1 sec = tan2 sec2 = sin2 cos2 cos2 = sin2 Example 1 Simplify the expression sin 1cos 1cos sin Solution Taking common denominator and using the identity cos2 sin2 = 1 we nd 2To integrate tan^22x, also written as ∫tan 2 2x dx, tan squared 2x, (tan2x)^2, and tan^2(2x), we start by utilising standard trig identities to change the form of the integral Our goal is to have sec 2 2x in the new form because there is a standard integration solution for that in formula booklets that we can use We recall the Pythagorean trig identity, and multiply the angles by 2
Prove Tan 2 Cot 2 Sec 2 Cosec 2 2 Brainly In
Prove The Following Identities 1 Tanatanb 2 Tan A Tan B 2 S
Apr 07, 16 · Get an answer for 'How do you prove the identity `(tanxcotx)^2=sec^2x csc^2x ?` ``' and find homework help for other Math questions at eNotesJan 09, 16 · A follow up proof to accompany sin^2 cos^2 =1 Another identity that is used quite a bit, especially in calculus involving trigonometric functionsJan 21, · In the second method, we used the identity \({\sec}^2 \theta={\tan}^2 \theta1\) and continued to simplify This problem illustrates that there are multiple ways we can verify an identity Employing some creativity can sometimes simplify a procedure As long as the substitutions are correct, the answer will be the same
Bestmaths Online Proof 4
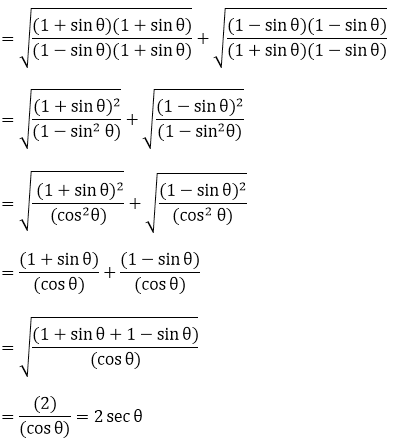
R D Sharma Class 10 Solutions Maths Chapter 6 Trigonometric Identities Exercise 6 1
1tan 2 a = sec 2 a As it is known that tan a is not defined for a = 90° therefore identity 2 obtained above is true for 0 ≤ AQuestion Prove the identity tan(2) sec?Yes, sec 2 x−1=tan 2 x is an identity sec 2 −1=tan 2 x Let us derive the equation We know the identity sin 2 (x)cos 2 (x)=1 ——(i) Dividing throughout the equation by cos 2 (x) We get sin 2 (x)/cos 2 (x) cos 2 (x)/cos 2 (x) = 1/cos 2 (x) We know that sin 2 (x)/cos 2 (x)= tan 2 (x), and cos 2 (x)/cos 2 (x) = 1 So the equation (i) after substituting becomes
Solved 2 Prove The Following Trig Identities A Prove Chegg Com
Prove That Math Tan 2 Theta 1 Sec 2 Theta Math Quora
Establish each identity $$9 \sec ^{2} \theta5 \tan ^{2} \theta=54 \sec ^{2} \theta$$ Answer View Answer Topics Trigonometry Functions Precalculus 9th Chapter 7 Analytic Trigonometry Section 4 Trigonometric Identities Discussion You must beLegend x and y are independent variables, ;Nov 06, 15 · Get an answer for '`cot^2(y)(sec^2(y) 1) = 1` Verfiy the identity' and find homework help for other Math questions at eNotes
Prove The Identity Tan P 4 X Tan P 4 X 2 Sec 2x Sarthaks Econnect Largest Online Education Community
Tan2 1 Proove Sec Tan3cosec 2 3 2 Maths Trigonometric Identities Meritnation Com
Dec 03, · Is \(\tan ^{2} x\cot ^{2} x=1\) a legitimate identity?Prove the following trigonometric identity $$1 \tan^2\theta = \sec^2\theta$$ I'm curious to know of the different ways of proving this depending on different characterizations of tangent and secant trigonometry alternativeproof Share Cite Follow edited Dec 16 '13 at 143The Pythagorean formula for sines and cosines sin 2 t cos 2 t = 1 Identities expressing trig functions in terms of their complements cos t = sin(/2 – t) sin t = cos(/2 – t) cot t = tan(/2 – t) tan t = cot(/2 – t) csc t = sec(/2 – t) sec t = csc(/2 – t) Periodicity of trig functions
Omtex Classes Prove The Identity Sin8 Cosec8 2 Cosec8 Sec8 2 7 Tan 2 8 Cot 2 8
Demo Identite 1 Tan2 Sec2 Youtube
The Pythagorean identity of secant and tan functions can also be written popularly in two other forms $\sec^2{x}\tan^2{x} \,=\, 1$ $\sec^2{A}\tan^2{A} \,=\, 1$ Remember, the angle of a right triangle can be represented by any symbol but the relationship between secant and tan functions must be written in that symbolFeb 26, 15 · Trigonometric Identities Basic Definitions Definition of tangent $ \tan \theta = \frac{\sin \theta}{\cos\theta} $ Definition of cotangent $ \cot \theta = \frac{\cosMath 172 Trigonometric Integrals Section 72 Done Right 1 Integrals of the form Z sinm cosn d If the power of cosine is odd and positive, save one cosine factor, use cos2 = 1 sin2 to express the remaining factors in terms of sine, and substitute u= sin so that du= cos d
Pre Calculus Math 40s Trigonometry Ii Lesson 2
How I Remember Trig Identities Part 2 Beyond Solutions
D is the differential operator, int is the integration operator, C is the constant of integration Identities tan x = sin x/cos x equation 1 cot x = cos x/sin x equation 2 sec x = 1/cos x equation 3 csc x = 1/sin x equation 4Trigonometric identities are equations that relate different trigonometric functions and are true for any value of the variable that is there in the domainBasically, an identity is an equation that holds true for all the values of the variable(s) present in itIn mathematics, trigonometric substitution is the substitution of trigonometric functions for other expressions In calculus, trigonometric substitution is a technique for evaluating integralsMoreover, one may use the trigonometric identities to simplify certain integrals containing radical expressions Like other methods of integration by substitution, when evaluating a definite integral, it
Integrate Sec 2x Method 2
Sample Problems Cos 2 X Tan2 X Tan 2 Csc 2 Tan Sec X Tan X Cos X Sin 4 X Cos 4 X 1 2 Cos 2 X Pdf Free Download
Name _____ ID A 2 ____ 8 Write the expression csc cot sin cos as a single term A csc C sin B sec D tan ____ 9 What are the nonpermissible values of for tan ?Identities In this unit we are going to look at trigonometric identities and how to use them to solve tan2 A1=sec2 A Thisisanotherimportantidentity Key Point tan2 A1=sec2 A Onceagain,returningto sin 2Acos A =1 wecandividethroughbysin2 A togive sin2 A sin 2A cos2 A sin A = 1 sin2 A ButTrigonometric Identities This is a list of the basic trigonometric identities You may want to work through a tutorial, Review of Trigonometric Identities Pythagoren Identities sin 2 x cos 2 x = 1 tan 2 x 1 = sec 2 x 1 cot 2 x = csc 2 x Reciprocal Identities csc x = 1 / sin x sec x = 1 / cos x cot x = 1 / tan x The Tangent and
Pin On Math Videos
Sample Problems Cos 2 X Tan2 X Tan 2 Csc 2 Tan Sec X Tan X Cos X Sin 4 X Cos 4 X 1 2 Cos 2 X Pdf Free Download
Nov 09, · Sine, tangent, cotangent and cosecant in mathematics an identity is an equation that is always true Meanwhile trigonometric identities are equations that involve trigonometric functions that are always trueThe provided equation is an identity but there are no steps available (tan(x)cot(x))2 = sec2 (x)csc2(x) (tan (x) cot (x)) 2 = sec 2 (x) csc 2 (x) is an identityThe answer is ' tan 2 θ tan 2 θ ' This is derived from the Pythagorean trigonometric identity tan 2 θ 1 = sec 2 θ tan 2 θ 1 = sec 2 θ What is the value of csc 2 θ − cot 2 θ csc 2 θ cot 2 θ ?
Trigonometric Identities A Plus Topper
Ch Ppt Download
Ask for it or check my other videos and playlists!##### PLAYLISTS #####Sec 2 A cosec 2 A = tan 2 A cot 2 A 2 Advertisement Remove all ads Solution Show Solution LHS = sec 2 A cosec 2 A = `1/(cos^2Asin^2A)` Chapter 21 Trigonometrical Identities Exercise 21 (A) Q 22 Page 324 Video Tutorials VIEW ALL 5 view Video Tutorials For All Subjects ;Pythagorean Identities The proof of the Pythagorean identity for sine and cosine is essentially just drawing a right triangle in a unit circle, identifying the cosine as the \(x\) coordinate, the sine as
Rd Sharma Solutions For Class 10 Chapter 6 Trigonometric Identities Exercise 6 1 Get Pdf
Trigonometric Identities
Trig Identity Sec2x Minus Tan2x T10 Youtube
How Do You Simplify Sec 4x Tan 4x Sec 2x Tan 2x Socratic
Trigonometric Identities List Of Trigonometric Identities Examples
Solved Verify The Identity 4 2 Tan X Secx2tan X 1 Whic Chegg Com
Trigonometric Identities Simplify Expressions Video Lessons Examples And Solutions
Kpk8sizotftvlm
Trigonometry Reciprocal Identities Expii
Geneseo Math 222 01 Trigonometric Integration
Warm Up Prove Sin 2 X Cos 2 X 1 This Is One Of 3 Pythagorean Identities That We Will Be Using In Ch 11 The Other 2 Are 1 Tan 2 X Sec 2 X Ppt Download
Which Of The Following Expressions Completes The Identity 1 Sec 2 X Mathematics Stack Exchange
Tangent Half Angle Formula Wikipedia
Prove The Identity Tan P 4 X Tan P 4 X 2 Sec 2x Sarthaks Econnect Largest Online Education Community
Ppt Analytic Trig Powerpoint Presentation Free Download Id
5 1 Fundamental Trig Identities Sin 1cos 1tan 1 Csc Sec Cot Csc 1sec 1cot 1 Sin Cos Ppt Download
How Do You Simplify 1 Tan 2 X 1 Tan 2 X Socratic
Prove That Tantheta Cottheta 2 Sec 2 Theta Cosec 2 Theta
Cbse 10 Math Cbse Introduction To Trigonometry Ncert Solutions
Which Of The Following Four S See How To Solve It At Qanda
Prove Sec 2 Theta 1 Tan 2 Theta
Integrate Sec 2x Method 2
Integrate Sec 2x Method 2
List Of Trigonometric Identities Wikipedia
Brandi S Buzzar Blog Proof Derivative Tan X Sec 2 X
Get Answer Prove The Given Identity Cos 2 Theta 1 Tan2 Theta 1 Which Transtutors
Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo
Solved 31 Proving Identities Verify The Identi
Trigonometric Identities List Of Trigonometric Identities Examples
14 2 Trigonometric Identities
Answered Verify The Identity 2 Sec T Sec Tcsc T Bartleby
Cos2x Sin2x 1 1 Tan2x Sec2x Cot2x 1 Csc2x Cofunction
Tan 2 X Tan 2 X 1 Cosec 2 X Sec 2 X Cosec 2 X 1 Sin 2 X Cos 2 X Brainly In
Trigonometric Identities A Plus Topper
How Do You Prove The Identities Cosx Secx Sinx Cscx Sec 2x Tan 2x Socratic
x-1=sec(squared)x.jpg "10 Identity Tan Squared X 1 Sec Squared X Trigonometry Educator Com")
10 Identity Tan Squared X 1 Sec Squared X Trigonometry Educator Com
Prove The Identity Sec 4 Theta Sec 2 Theta Tan 4 Theta Tan 2
.JPG "Every Day I M Calculatin I D3 Unit Q Pythagorean Identities")
Every Day I M Calculatin I D3 Unit Q Pythagorean Identities
Solved 31 Prove That Tan 2 Theta 1 Sec 2 Theta 32 Chegg Com
Solved Verify The Identity 2 X Tan 2 X Esec X Tan X Sec Chegg Com
Prove That Math Tan 2 Theta 1 Sec 2 Theta Math Quora
Solved Prove The Following Trig Identity Sec 2 X 2secx Cosx Cos 2 X Tan 2 X Sin 2 X Course Hero
Identities S Cool The Revision Website
To Prove Sec 4 8 Sec 2 8 Tan 4 8 Tan 2 8 Sarthaks Econnect Largest Online Education Community
Evaluating Trig Integral Int Tan 3x Sec 2x Dx Mathematics Stack Exchange
Trigonometric Substitution
Prove The Following Statement Sec 2a Cosec 2a Tan 2a Cot 2a 2
Prove That Tan2 Cot2 2 Sec2 Cosec2 Mathematics Topperlearning Com T5kfk8obb
Trig Identities Crossword Wordmint
Integrate Sec 2x Method 1
Solution Prove The Identity Cos X 1 Sin X Cos X 1 Sin X 2 1 Tan 2 X Sec X
Trig Identities Hsn Forum
Integration Calculus 2
Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo
Proof Tan 2 1 Sec 2 Youtube
Solved Trig Identities 1 Sin Cos 2 Sin Cos 2 2 2 Tan2 Cos2 Cot2 Sin2 1 3 Sec Course Hero
Prove That Square Root Of Sec 2 Theetah Cosec 2 Theetah Tan Theetah Cot Theetah Mathematics Topperlearning Com Fpq77bww
11 Basic Trigonometric Identities An Identity Is An Equation That Is True For All Defined Values Of A Variable We Are Going To Use The Identities To Ppt Download
How Do You Prove Tan 2x Secx 1 1 Secx Socratic
Proving Trigonometric Identities
Prove That Tan 2 Theta Cot 2theta 2 Sec 2theta Cosec 2theta Brainly In
5 1 5 2 Trigonometric Identities Ppt Download
Find The General Solution Sec22x 1 Tan 2x Maths Trigonometric Functions Meritnation Com
Rd Sharma Solutions For Class 10 Chapter 6 Trigonometric Identities Exercise 6 1 Get Pdf
2 Sec 0 1 Tan E 1 1 0 2 2 3 3 4 4
Sec Squared Theta Minus Sin Squared Theta Minus Two Sine Power Four 2 Cos Power 4 Cos Squared 1
View Question For Questions 1 5 Decide Whether The Equation Is A Trigonometric Identity Explain Your Reasoning
Solving Trigonometric Equations
Powers Of Trigonometric Functions
11 X1 T04 03 Pythagorean Trig Identities 10
Verifying Trigonometric Identities Process Make One Side Look
Trigonometry Identity Tan 2 X 1 Sec 2 X Youtube
Which Of The Following Is A Trigonometric Identity Gauthmath
Ppt 5 1 Fundamental Trig Identities Powerpoint Presentation Free Download Id
Solving Trigonometric Equations With Identities Algebra And Trigonometry
Using Trigonometric Identities Video Khan Academy
0 件のコメント:
コメントを投稿